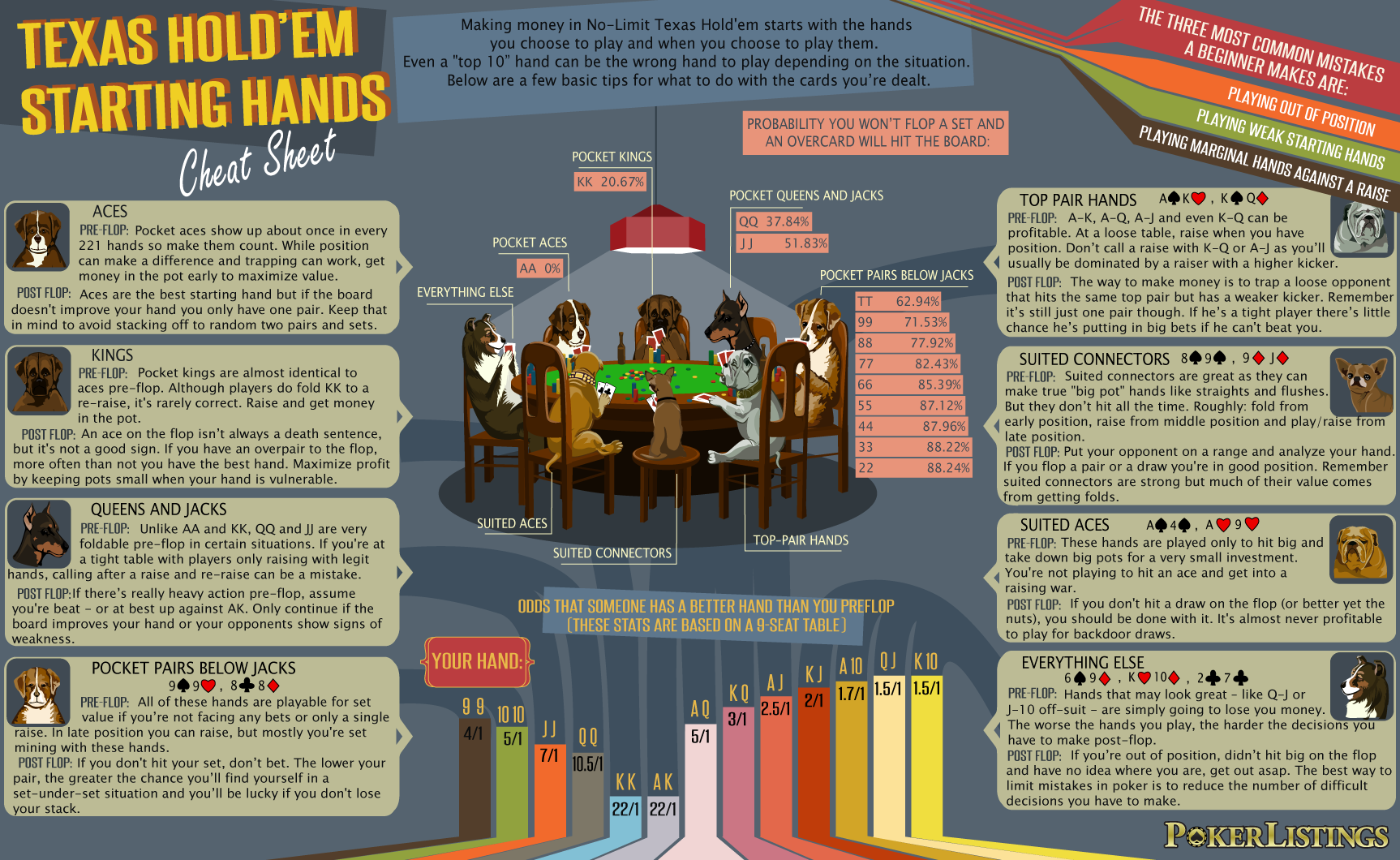
Every Poker Hand
“Fast-fold” poker has become an indispensable part of anyone’s online poker real money portfolio. Instead of playing, say, 100 hands per hour at a cash table, you can double or even triple that volume by playing Zone Poker. As soon as you fold a hand, you’ll be whisked away to an open seat with fresh hole cards waiting for you. Autoplay is paused. You're signed out. Videos you watch may be added to the TV's watch history and influence TV recommendations. To avoid this, cancel and sign in to YouTube on your computer. On this site you can find all possible combinations of preflop hands that can occur in Texas Hold'em Poker. As a bonus you will also learn the nicknames of the different hands. The hands are ranked from #1 to #169, where #1 is the best. This ranking is applicable when the poker table is full ring (9-10 people). The is the best possible hand you can get in standard five-card Poker is called a royal.
POKER PROBABILITIES
- Texas Hold'em Poker
Texas Hold'em Poker probabilities - Omaha Poker
Omaha Poker probabilities - 5 Card Poker
5 Card Poker probabilities
POKER CALCULATOR

- Poker calculator
Poker odds calculator
POKER INFORMATION
- Poker hand rankings
Ranking of poker hands
In poker, the probability of each type of 5-card hand can be computed by calculating the proportion of hands of that type among all possible hands.
Frequency of 5-card poker hands
The following enumerates the (absolute) frequency of each hand, given all combinations of 5 cards randomly drawn from a full deck of 52 without replacement. Wild cards are not considered. The probability of drawing a given hand is calculated by dividing the number of ways of drawing the hand by the total number of 5-card hands (the sample space, five-card hands). The odds are defined as the ratio (1/p) - 1 : 1, where p is the probability. Note that the cumulative column contains the probability of being dealt that hand or any of the hands ranked higher than it. (The frequencies given are exact; the probabilities and odds are approximate.)
The nCr function on most scientific calculators can be used to calculate hand frequencies; entering nCr with 52 and 5, for example, yields as above.
| Hand | Frequency | Approx. Probability | Approx. Cumulative | Approx. Odds | Mathematical expression of absolute frequency |
|---|---|---|---|---|---|
| Royal flush | 4 | 0.000154% | 0.000154% | 649,739 : 1 | |
| Straight flush (excluding royal flush) | 36 | 0.00139% | 0.00154% | 72,192.33 : 1 | |
| Four of a kind | 624 | 0.0240% | 0.0256% | 4,164 : 1 | |
| Full house | 3,744 | 0.144% | 0.170% | 693.2 : 1 | |
| Flush (excluding royal flush and straight flush) | 5,108 | 0.197% | 0.367% | 507.8 : 1 | |
| Straight (excluding royal flush and straight flush) | 10,200 | 0.392% | 0.76% | 253.8 : 1 | |
| Three of a kind | 54,912 | 2.11% | 2.87% | 46.3 : 1 | |
| Two pair | 123,552 | 4.75% | 7.62% | 20.03 : 1 | |
| One pair | 1,098,240 | 42.3% | 49.9% | 1.36 : 1 | |
| No pair / High card | 1,302,540 | 50.1% | 100% | .995 : 1 | |
| Total | 2,598,960 | 100% | 100% | 1 : 1 |
How To Play Every Poker Hand
The royal flush is a case of the straight flush. It can be formed 4 ways (one for each suit), giving it a probability of 0.000154% and odds of 649,739 : 1.
When ace-low straights and ace-low straight flushes are not counted, the probabilities of each are reduced: straights and straight flushes each become 9/10 as common as they otherwise would be. The 4 missed straight flushes become flushes and the 1,020 missed straights become no pair.
Note that since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. For example, the hand 3♣ 7♣ 8♣ Q♠ A♠ is identical to 3♦ 7♦ 8♦ Q♥ A♥ because replacing all of the clubs in the first hand with diamonds and all of the spades with hearts produces the second hand. So eliminating identical hands that ignore relative suit values, there are only 134,459 distinct hands.
How To Play Every Poker Hand
The number of distinct poker hands is even smaller. For example, 3♣ 7♣ 8♣ Q♠ A♠ and 3♦ 7♣ 8♦ Q♥ A♥ are not identical hands when just ignoring suit assignments because one hand has three suits, while the other hand has only two—that difference could affect the relative value of each hand when there are more cards to come. However, even though the hands are not identical from that perspective, they still form equivalent poker hands because each hand is an A-Q-8-7-3 high card hand. There are 7,462 distinct poker hands.
Derivation of frequencies of 5-card poker hands
of the binomial coefficients and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory).
- Straight flush — Each straight flush is uniquely determined by its highest ranking card; and these ranks go from 5 (A-2-3-4-5) up to A (10-J-Q-K-A) in each of the 4 suits. Thus, the total number of straight flushes is:
- Royal straight flush — A royal straight flush is a subset of all straight flushes in which the ace is the highest card (ie 10-J-Q-K-A in any of the four suits). Thus, the total number of royal straight flushes is
- or simply . Note: this means that the total number of non-Royal straight flushes is 36.
- Royal straight flush — A royal straight flush is a subset of all straight flushes in which the ace is the highest card (ie 10-J-Q-K-A in any of the four suits). Thus, the total number of royal straight flushes is
- Four of a kind — Any one of the thirteen ranks can form the four of a kind by selecting all four of the suits in that rank. The final card can have any one of the twelve remaining ranks, and any suit. Thus, the total number of four-of-a-kinds is:
- Full house — The full house comprises a triple (three of a kind) and a pair. The triple can be any one of the thirteen ranks, and consists of three of the four suits. The pair can be any one of the remaining twelve ranks, and consists of two of the four suits. Thus, the total number of full houses is:
- Flush — The flush contains any five of the thirteen ranks, all of which belong to one of the four suits, minus the 40 straight flushes. Thus, the total number of flushes is:
- Straight — The straight consists of any one of the ten possible sequences of five consecutive cards, from 5-4-3-2-A to A-K-Q-J-10. Each of these five cards can have any one of the four suits. Finally, as with the flush, the 40 straight flushes must be excluded, giving:
- Three of a kind — Any of the thirteen ranks can form the three of a kind, which can contain any three of the four suits. The remaining two cards can have any two of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of three-of-a-kinds is:
- Two pair — The pairs can have any two of the thirteen ranks, and each pair can have two of the four suits. The final card can have any one of the eleven remaining ranks, and any suit. Thus, the total number of two-pairs is:
- Pair — The pair can have any one of the thirteen ranks, and any two of the four suits. The remaining three cards can have any three of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of pair hands is:
- No pair — A no-pair hand contains five of the thirteen ranks, discounting the ten possible straights, and each card can have any of the four suits, discounting the four possible flushes. Alternatively, a no-pair hand is any hand that does not fall into one of the above categories; that is, any way to choose five out of 52 cards, discounting all of the above hands. Thus, the total number of no-pair hands is:
- Any five card poker hand — The total number of five card hands that can be drawn from a deck of cards is found using a combination selecting five cards, in any order where n refers to the number of items that can be selected and r to the sample size; the '!' is the factorial operator:
This guide is licensed under the GNU Free Documentation License. It uses material from the Wikipedia.
Home > 5 Card Poker probabilities
Hand Guide: Preflop > Flop > Turn > River
The Texas Holdem hand guide below will take you through each stage of a poker hand from the preflop round to the river, and give you a brief overview of the strategy you should try and employ as well as the thought processes behind making profitable decisions at each stage of the hand.
Knowing how to play a poker hand well from start to finish is not an easy skill to learn. Many players can play certain rounds well, but struggle on others. It is important to learn how to play each round profitably.
Win Percentage Of Every Poker Hand
The individual Texas Holdem strategy articles found on this site will help to fine tune different aspects of your game, but it is here that you will find the fundamental principles behind playing a winning Texas Holdem hand. There is also a list of useful articles at the end of each guide for further information on different concepts and plays that you can use at each stage in the hand.
Individual betting round strategy articles.
Before the flop is where it all starts, so learn how to get the foundations started correctly. This article focuses on good starting hand selection, along with key position tips and a quick guide to optimum preflop betting.
Many of the decisions that you will make at the poker table will be based on the flop or will stem from the flop later in the hand. This article highlights the importance of being able to identify the different textures of flop and the best strategies for dealing with them.
The turn is often forgotten about in the Texas Holdem strategy world but can often prove to be a very important street. So don't forget about it and learn how to play it well.
Now all of the cards are out and its time to make the best 5-card hand possible. Pick up some key tips on how to play when you have the best of it and when you have the worst of it.
Playing a good Poker hand is not always easy, as there are always going to be opportunities to make mistakes along the way. Use the tips in this article to help yourself make more profitable decisions on each betting round.
Bonus Article:Poker Mistakes - Avoid the most common mistakes when playing a hand.
Playing a hand in Texas Hold'em.
These articles are quite lengthy, but they are actually as condensed as I could make them without leaving out any tips or strategy that I thought was vital to being able to play each betting round successfully. However, although these articles should prove to be a handy guide for you, you should try your best to learn from them, and not rely on using them every time you make a decision at the Texas Hold'em poker table.
Try not to refer to these guides too often whilst playing poker. Learn from these articles as best as you can and use your own judgment when making decisions at the poker table.
Being able to stand on your own two feet and make decisions for yourself is what is going to help you develop into the best player that you can be. So be prepared to eventually move away from using guides, and try to use your head as much as you can during play. Ask yourself questions like:
Chances Of Every Poker Hand

- What do I expect to achieve with this play?
- What am I going to do on the next betting round if I make this play?
- How do I think my opponent is going to react to this play?
The more questions you ask yourself, the more refined and calculated your plays will be. A winning poker player is a thinking poker player, so you're going to have to use that logic of yours if you want to win money. If you do not use your head, you may as well play online casino games instead.
Poker is a game of skill, so use your ability to get the better of your opponents.